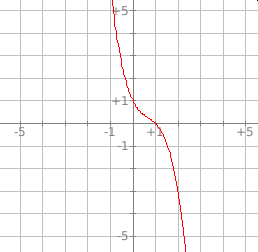
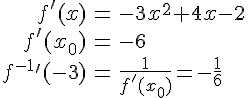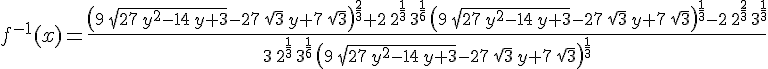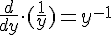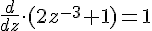Opgaver Differantial

Billede:Copy icon.png

Aflevering

Denne side er en aflevering og delene må

kun afleveres af deres respektive ejere.

Indholdsfortegnelse

Uge 3 (Kap. 2)

226

Viser at linjen er en tangent.
Forstør
Viser at linjen er en tangent.

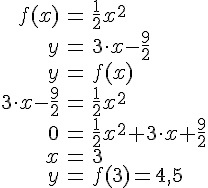
LaTex: \begin{eqnarray} f(x) &=& \frac{1}{2}x^2\\ y &=& 3\cdot x -\frac{9}{2}\\ y &=& f(x)\\ 3\cdot x -\frac{9}{2} &=& \frac{1}{2}x^2\\ 0 &=& \frac{1}{2}x^2 + 3\cdot x +\frac{9}{2}\\ x &=& 3\\ y &=& f(3) = 4,5 \end{eqnarray}

231

Forstør

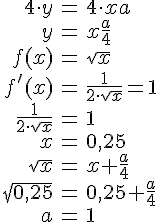
LaTex: \begin{eqnarray} 4\cdot y &=& 4\cdot x a\\ y &=& x \frac{a}{4}\\ f(x) &=& \sqrt{x}\\ f'(x) &=& \frac{1}{2\cdot \sqrt{x}} = 1\\ \frac{1}{2\cdot \sqrt{x}} &=& 1\\ x &=& 0,25\\ \sqrt{x} &=& x + \frac{a}{4}\\ \sqrt{0,25} &=& 0,25 + \frac{a}{4}\\ a &=& 1\\ \end{eqnarray}

f'(x) = 1 da hældnings graden for tangenten er 1.

250

Opg 1
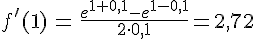
LaTex: \begin{eqnarray} f'(1) &=& \frac{e^{1+0,1}-e^{1-0,1}}{2\cdot 0,1} = 2,72\\ \end{eqnarray}
Opg 3
LaTex: \begin{eqnarray} f'(\pi) &=& \frac{sin(\pi + 0,1)-sin(\pi-0,1)}{2\cdot 0,1} = -0.998\\ \end{eqnarray}

257

Forstør

LaTex: \begin{eqnarray} y &=& 2\cdot x - 2\\ 4\cdot x - 8\cdot y +1 &=& 0\\ 8\cdot y &=& 4\cdot x +1 \\ y &=& \frac{1}{2}\cdot x +\frac{1}{8} \\ y &=& -\frac{1}{2}\cdot x^2\\ -2\cdot \frac{1}{2} &=& -1\\ 0 &=& -\frac{1}{2}\cdot x^2+2\cdot x -2\\ x &=& 2\\ y &=& -\frac{1}{2}\cdot 2^2 = -2\\ 0 &=& -\frac{1}{2}\cdot x^2-\frac{1}{2}\cdot x - \frac{1}{8}\\ x &=& -\frac{1}{2}\\ y &=& -\frac{1}{2}\cdot \left(-\frac{1}{2}\right)^2 = -\frac{1}{8}\\ \end{eqnarray}

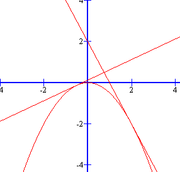
De er ortogonale, da produktet af deres hældninger giver -1.

Uge 4 (Kap. 3)

311

1
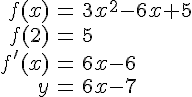
LaTex: \begin{eqnarray}f(x) &=& 3x^2-6x+5\\f(2) &=& 5\\f'(x) &=& 6x-6\\y &=& 6x-7\end{eqnarray}
2
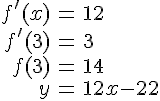
LaTex: \begin{eqnarray}f'(x) &=& 12\\f'(3) &=& 3\\f(3) &=& 14\\y &=& 12x-22\\\end{eqnarray}
3
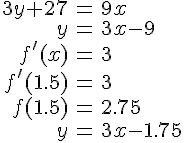
LaTex: \begin{eqnarray}3y+27 &=& 9x\\y &=& 3x-9\\f'(x) &=& 3\\f'(1.5) &=& 3\\f(1.5) &=& 2.75\\y &=& 3x-1.75\\\end{eqnarray}

318

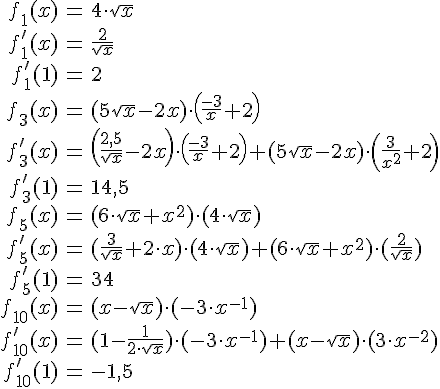
LaTex: \begin{eqnarray} f_1(x) &=& 4\cdot \sqrt{x}\\ f_1'(x) &=& \frac{2}{\sqrt{x}}\\ f_1'(1) &=& 2\\ f_3(x) &=& (5\sqrt{x}-2x)\cdot\left(\frac{-3}{x}+2\right)\\ f_3'(x) &=& \left(\frac{2,5}{\sqrt{x}}-2x\right)\cdot\left(\frac{-3}{x}+2\right)+(5\sqrt{x}-2x)\cdot\left(\frac{3}{x^2}+2\right)\\ f_3'(1) &=& 14,5\\ f_5(x) &=& (6\cdot\sqrt{x}+x^2)\cdot (4\cdot \sqrt{x})\\ f_5'(x) &=& (\frac{3}{\sqrt{x}}+2\cdot x)\cdot (4\cdot\sqrt{x})+(6\cdot\sqrt{x}+x^2)\cdot (\frac{2}{\sqrt{x}})\\ f_5'(1) &=& 34\\ f_{10}(x) &=& (x-\sqrt{x})\cdot (-3\cdot x^{-1})\\ f_{10}'(x) &=& (1-\frac{1}{2\cdot\sqrt{x}})\cdot (-3\cdot x^{-1}) + (x-\sqrt{x})\cdot (3\cdot x^{-2})\\ f_{10}'(1) &=& -1,5\\ \end{eqnarray}

325

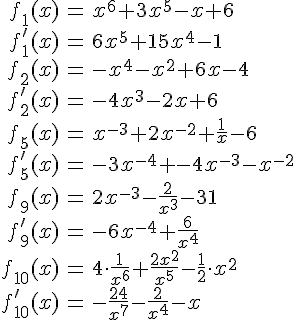
LaTex: \begin{eqnarray} f_1(x)&=&x^6+3x^5-x+6\\ f_1'(x)&=&6x^5+15x^4-1\\ f_2(x)&=&-x^4-x^2+6x-4\\ f_2'(x)&=&-4x^3-2x+6\\ f_5(x)&=&x^{-3}+2x^{-2}+\frac{1}{x}-6\\ f_5'(x)&=&-3x^{-4}+-4x^{-3}-x^{-2}\\ f_9(x)&=&2x^{-3}-\frac{2}{x^3}-31\\ f_9'(x)&=&-6x^{-4}+\frac{6}{x^4}\\ f_{10}(x)&=&4\cdot\frac{1}{x^6}+\frac{2x^2}{x^5}-\frac{1}{2}\cdot x^2\\ f_{10}'(x)&=&-\frac{24}{x^7}-\frac{2}{x^4}-x\\ \end{eqnarray}

330

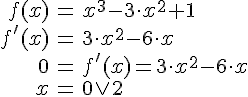
LaTex: \begin{eqnarray} f(x) &=& x^3-3\cdot x^2 +1\\ f'(x) &=& 3\cdot x^2 -6\cdot x\\ 0 &=& f'(x) = 3\cdot x^2 -6\cdot x\\ x &=& 0 \vee 2\\ \end{eqnarray}

346

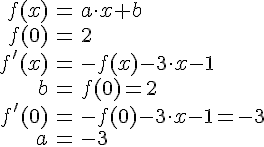
LaTex: \begin{eqnarray} f(x) &=& a\cdot x + b\\ f(0)&=& 2\\ f'(x) &=& -f(x) -3\cdot x -1\\ b &=& f(0) = 2\\ f'(0) &=& -f(0) -3\cdot x -1 = -3\\ a &=& -3\\ \end{eqnarray}

350

  1. LaTex: \frac{d(x^3+2x)}{dx}=3x^2+2
  2. LaTex: \frac{d(x^2+2x-1)}{dx}=2x+2
  3. LaTex: \frac{d(t^2+2\sqrt{t})}{dt}=2t+\sqrt{t}^{-1}
  4. LaTex: \frac{d}{dy}\cdot(2x+1)=1
  5. LaTex: \frac{d}{dy}\cdot(\frac{1}{y})=y^{-1}
  6. LaTex: \frac{d}{dz}\cdot(2z^{-3}+1)=1

355

LaTex: \begin{eqnarray} f_1(x)&=&\\ f_1'(x)&=&\\ f_1''(x)&=&\\ f_4(x)&=&\\ f_4'(x)&=&\\ f_4''(x)&=&\\ f_7(x)&=&\\ f_7'(x)&=&\\ f_7''(x)&=&\\ f_9(x)&=&\\ f_9'(x)&=&\\ f_9''(x)&=&\\ \end{eqnarray}

370

LaTex: \begin{eqnarray} f(x) &=& \sqrt{x-3}\\ f(x_0) &=& \sqrt{x_0-3} = 2\\ y_0 &=& 2\\ x_0 &=& y_0^2+3 = 7\\ f'(x) &=& \frac{1}{2\cdot \sqrt{x-3}}\\ a_{tan} &=& f'(x_0) = 0,25\\ b_{tan} &=& y_0-a_{tan}\cdot x_0 = 0,25\\ f_{tan}(x) &=& a_{tan}\cdot x +b_{tan}\\ y &=& f_{tan}(7) = 2\\ f_{tan}(x_1) &=& 0 = a_{tan}\cdot x_1 +b_{tan}\\ x_1 &=& -\frac{b_{tan}}{a_{tan}} = -1\\ Areal &=& \frac{1}{2}\cdot(y_0-0)\cdot (7-x_1) = 8\\ \end{eqnarray}

372

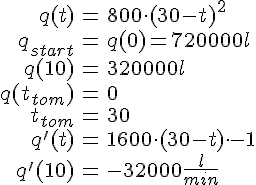
LaTex: \begin{eqnarray} q(t) &=& 800\cdot (30-t)^2\\ q_{start} &=& q(0) = 720000 l\\ q(10) &=& 320000 l\\ q(t_{tom}) &=& 0\\ t_{tom} &=& 30\\ q'(t) &=& 1600\cdot (30-t)\cdot -1\\ q'(10) &=& -32000 \frac{l}{min} \end{eqnarray}

Uge 5 (Kap. 4)

401

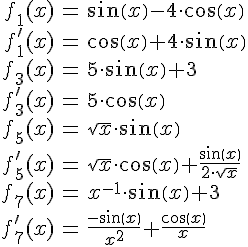
LaTex: \begin{eqnarray} f_1(x) &=& sin(x)-4\cdot cos(x)\\ f_1'(x) &=& cos(x)+4\cdot sin(x)\\ f_3(x) &=& 5\cdot sin(x) +3\\ f_3'(x) &=& 5\cdot cos(x)\\ f_5(x) &=& \sqrt{x}\cdot sin(x)\\ f_5'(x) &=& \sqrt{x}\cdot cos(x) + \frac{sin(x)}{2\cdot sqrt{x}}\\ f_7(x) &=& x^{-1}\cdot sin(x)+3\\ f_7'(x) &=& \frac{-sin(x)}{x^2}+\frac{cos(x)}{x}\\ \end{eqnarray}

403

LaTex: \begin{eqnarray} f_1(x) &=& (2\cdot x + sin(x))\cdot (x-cos(x))\\ f_1'(x) &=& (2+cos(x))\cdot (x-cos(x))+(2\cdot x + sin(x))\cdot (1+sin(x))\\ f_1'(0) &=& -3\\ f_3(x) &=& (3\cdot sin(x) + 5)\cdot (x-4)\\ f_3'(x) &=& (3\cdot cos(x))\cdot (x-4) + (3\cdot sin(x) + 5)\cdot 1\\ f_3'(0) &=& -7\\ \end{eqnarray}

406

410

LaTex: \begin{eqnarray} l_a:2y&=&3x+2a\\ y&=&1,5x+a\\ f(x)&=&2x+cos(x)+1\\ x&\in& ]-\frac{\pi}{2};\frac{\pi}{2}[\\ f'(x)&=&2-sin(x)\\ 1,5 &=& f'(x)\\ &\Downarrow&\\ x&=& sin^{-1}(0,5)=0,52\\ y&=&f(x)=2,91\\ a&=&y-1,5\cdot x=2,13 \end{eqnarray}

411

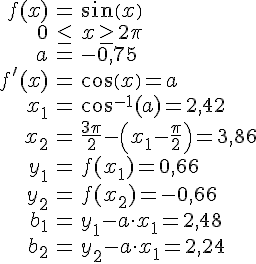
LaTex: \begin{eqnarray} f(x)&=&sin(x)\\ 0&\leq& x \geq 2\pi\\ a &=& -0,75 \\ f'(x)&=&cos(x)=a\\ x_1&=&cos^{-1}(a)=2,42\\ x_2&=&\frac{3\pi}{2}-\left(x_1-\frac{\pi}{2}\right)=3,86\\ y_1&=&f(x_1)=0,66\\ y_2&=&f(x_2)=-0,66\\ b_1&=&y_1 -a\cdot x_1=2,48\\ b_2&=&y_2-a\cdot x_1=2,24\\ \end{eqnarray}

418


Opgave 375

Opgave 1
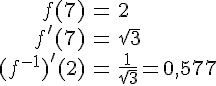
LaTex: \begin{eqnarray} f(7) &=& 2\\ f'(7) &=& \sqrt{3}\\ (f^{-1})'(2) &=& \frac{1}{\sqrt{3}} = 0,577\\ \end{eqnarray}
Opgave 2
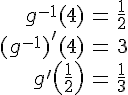
LaTex: \begin{eqnarray} g^{-1}(4) &=& \frac{1}{2}\\ (g^{-1})'(4) &=& 3\\ g'\left(\frac{1}{2}\right) &=& \frac{1}{3} \end{eqnarray}

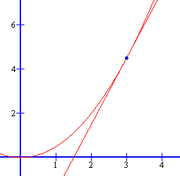
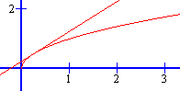
Opgave 376

Funktionen f(x) er injektiv, da der ikke findes 2 steder der har ens y/x-koordinater

f^-1(-3)
LaTex: \begin{eqnarray} f(x_0) &=& f(f^{-1}(-3)) = -3\\ -3 &=& -x_0^3+2\cdot x_0 ^2 - 2\cdot x_0 +1\\ x_0 &=& f^{-1}(-3) = 2\\ \end{eqnarray}
Da en inverse funktion ophæver den originale funktion, kan man løse opgaven på denne måde.
f^-1'(-3)
LaTex: \begin{eqnarray} f'(x) &=& -3x^2+4x-2\\ f'(x_0) &=& -6\\ f^{-1}'(-3) &=& \frac{1}{f'(x_0)} = -\frac{1}{6} \end{eqnarray}

LaTex: f^{-1}(x) = {{\left(9\,\sqrt{27\,y^2-14\,y+3}-27\,\sqrt{3}\,y+7\,\sqrt{3} \right)^{{{2}\over{3}}}+2\,2^{{{1}\over{3}}}\,3^{{{1}\over{6}}}\, \left(9\,\sqrt{27\,y^2-14\,y+3}-27\,\sqrt{3}\,y+7\,\sqrt{3}\right)^{ {{1}\over{3}}}-2\,2^{{{2}\over{3}}}\,3^{{{1}\over{3}}}}\over{3\,2^{ {\frac{1}{3}}}\,3^{{\frac{1}{6}}}\,\left(9\,\sqrt{27\,y^2-14\,y+3}- 27\,\sqrt{3}\,y+7\,\sqrt{3}\right)^{{\frac{1}{3}}}}}

Se Evt.

Hentet fra "http://jopsen.dk/wiki/Opgaver_Differantial/"